
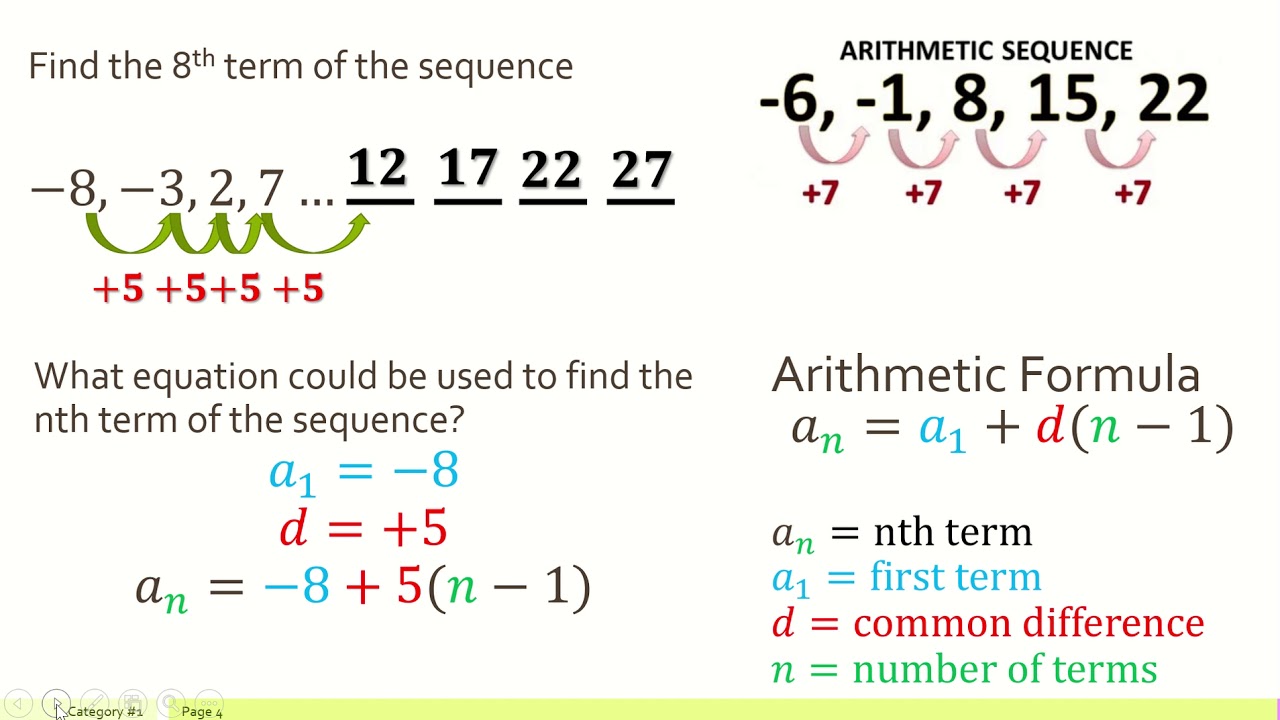
Further, an arithmetic sequence can be used find out savings, cost, final increment, etc. For an arithmetic sequence with first term u1 and common difference d, the nth term is un u1 + (n 1) d. Hence, with the above discussion, it would be clear that there is a huge difference between the two types of sequences. In each of these sequences, the difference between consecutive terms is constant, and so the sequence is arithmetic. The difference between consecutive terms, an an 1, is d, the common difference, for n greater than or equal to two. The infinite arithmetic sequences, diverge while the infinite geometric sequences converge or diverge, as the case may be. An arithmetic sequence is a sequence where the difference between consecutive terms is always the same.As against this, the variation in the elements of the sequence is exponential. In an arithmetic sequence, the variation in the members of the sequence is linear.When we add a finite number of terms in an arithmetic. As opposed to, geometric sequence, wherein the new term is found by multiplying or dividing a fixed value from the previous term. When a sequence of numbers is added, the result is known as a series.

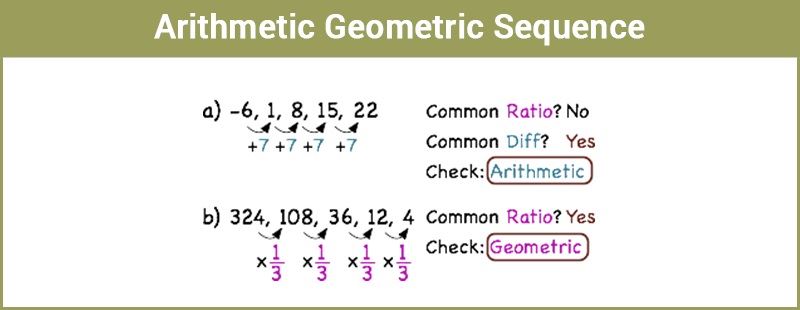
In an arithmetic sequence, the new term is obtained by adding or subtracting a fixed value to/from the preceding term.
#Geometric and arithmetic sequences equations how to#
We will also learn how to solve some practice problems. In this article, we will explore these sequences and learn how to write terms for both arithmetic and geometric sequences. On the contrary, when there is a common ratio between successive terms, represented by ‘r’, the sequence is said to be geometric. On the other hand, geometric sequences are formed by multiplying the terms by a common ratio.
A sequence can be arithmetic, when there is a common difference between successive terms, indicated as ‘d’.A set of numbers wherein each element after the first is obtained by multiplying the preceding number by a constant factor, is known as Geometric Sequence. As a list of numbers, in which each new term differs from a preceding term by a constant quantity, is Arithmetic Sequence.The following points are noteworthy so far as the difference between arithmetic and geometric sequence is concerned: Key Differences Between Arithmetic and Geometric Sequence Geometric Sequence is a set of numbers wherein each element after the first is obtained by multiplying the preceding number by a constant factor.Ĭommon Difference between successive terms. Content: Arithmetic Sequence Vs Geometric SequenceĪrithmetic Sequence is described as a list of numbers, in which each new term differs from a preceding term by a constant quantity. Here, in this article we are going to discuss the significant differences between arithmetic and geometric sequence. In an arithmetic sequence, the terms can be obtained by adding or subtracting a constant to the preceding term, wherein in case of geometric progression each term is obtained by multiplying or dividing a constant to the preceding term. We can see from the given explicit formula that \(r=2\).On the other hand, if the consecutive terms are in a constant ratio, the sequence is geometric. Find \(a_1\) by substituting \(k=1\) into the given explicit formula.


 0 kommentar(er)
0 kommentar(er)
